Use Number Talks to Boost Math Reasoning

By Cathy Humphreys & Ruth Parker

Most upper-grade teachers lament their students’ lack of mathematical understanding. They often secretly—or not so secretly—wonder what students did in their previous classes. Why don’t they know their multiplication facts in fourth, fifth, or even tenth grade? Why do some students still hide their fingers under the table as they count? And why do they have so much trouble with fractions?

The fact that this happens in so many classrooms across the country tells us that the failure lies not with the students or even their teachers, but in how mathematics has been taught, year after year, with the best of intentions.

Understanding quantities and numerical relationships is within the grasp of all students, yet many of them don’t realize it. Students come to classrooms fearing and avoiding math and, worse, thinking they are no good at it. Believing that mathematics is mostly about using procedures correctly, they have learned to focus on getting the right answer, whether or not the process makes sense to them.

Many students don’t expect math to make sense at all. The result is that students learn to disengage their reasoning—and even distrust it.

Number Talks can help students take back the authority of their own reasoning through a short, fifteen-minute daily routine, in which they reason mentally with numbers. Our purpose is to help teachers learn how to facilitate this routine so that, over time, students develop a strong sense of the meaning of quantities and operations while gaining proficiency with mathematical practices.

The good news is that Number Talks have a predictable structure that will support you in this rewarding path, no matter what grade you teach. The bad news is that there is not one set route to follow. We can’t tell you exactly what to do, and we wouldn’t want to even if we could!

The process of engaging students in reasoning with numbers is one we hope you will consider as a problem-solving venture—an investigation that will help you to learn to listen to your students and learn along with them as you build your lessons around their thinking.

Start making sense

We want to be crystal clear: teachers are not to blame for students’ general lack of deeper understanding about numbers. Most of us only know how to teach mathematics as we have been taught. And even when we try to change our practice, we often have found ourselves caught in a system whose demands run counter to the changes we had wanted to make.

The Standards for Mathematical Practice (NGA/CCSSO 2010) offer a new opportunity. With their focus on “attending to the meaning of quantities, not just how to compute them, and knowing and flexibly using different properties of operations and objects” (SMP2), these standards place mathematical sense-making squarely in the foreground of instruction.

Of course, students must be able to compute flexibly, efficiently, and accurately. But they also need to explain their reasoning and determine if the ideas they’re using and the results they’re getting make sense. And crucially, as Dr. Jo Boaler (2008) points out, our students also need to come to believe that these are the things they should always be doing in math class—because this is what mathematics is all about.

Students do make sense of computation during Number Talks. For example, here are some of the ways they solved 63 – 27 and the ways we recorded:

In contrast to traditional algorithms, Number Talks depend on students’ sense-making. They help students become confident mathematical thinkers more effectively than any single instructional practice we have ever used.

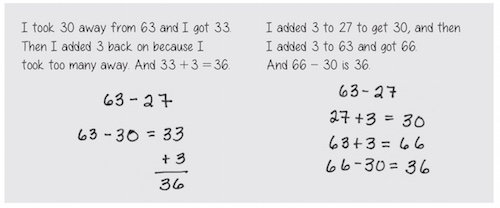
There are far too many students who feel like they are no good at math because they aren’t quick to get right answers. With Number Talks, students start to believe in themselves mathematically. They become more willing to persevere when solving complex problems. They become more confident when they realize that they have ideas worth listening to. And when students feel this way, the culture of a class can be transformed.

How to get started with number talks

The description that follows is as close to a recipe as you will find in our work. Each step in the routine has a rationale. (Note: Most teachers do Number Talks while students are at their regular desks, unless the classroom is large enough to gather students into a half circle, where they can leave their pencils behind and it can be easier to focus.)

1. Students put paper and pencils away (they may need reminding) and put their fists unobtrusively on their chests to show the teacher they are ready. This shifts students’ attention from working in groups and writing to thinking by themselves.

2. The teacher writes a problem on the board or document camera. Generally, we write problems horizontally to discourage the use of rote procedures.

3. The teacher watches while students solve the problem mentally and put up their thumbs when they have had enough time to think. Giving students whatever time they need is a powerful message about math that challenges the prevalent idea that being good at math means being fast. Also, how quickly—or not—thumbs come up is a good indication of a problem’s difficulty. Students who have extra time can be encouraged to solve the problem a second and even third way, and they indicate how many solutions they have by raising that number of fingers silently, so as not to interfere with the thinking of others.

4. When most thumbs are up, the teacher asks if anyone is willing to share what they think the answer is. She noncommittally records just the answer on the board and asks if anyone got a different answer, continuing to record each answer that is given. Students are asked not to indicate in any way whether they agree or disagree with any given answer; voting on answers has no place in mathematical discourse. You will find that some of the most productive Number Talks occur when students have suggested several different answers.

5. When the teacher is satisfied there are no other answers, she asks if anyone can explain how he or she figured the problem out. Describing the steps of a procedure is not enough; students need to be able to explain why their process makes sense. There are different ways we ask this question (see below). There is no “right” way, but here are two variations we find ourselves using frequently:

• Who has a strategy he or she is willing to share?
• Is anyone willing to convince us that your answer makes sense by telling us what you did?

6. When volunteers begin to share their strategies, they first identify which answer (assuming different answers have been offered) they are defending. Then, as they share their strategies, the teacher records the thinking of each student. (There are many examples of recording in Chapters 4–8.)

7. After a student shares a strategy, there are several things a teacher might ask in order to work with that student’s thinking. This is the tricky part, and, again, there is no one “right” question. The overall goal is to help the student communicate more clearly and/or to emphasize particular elements of his or her strategy.

• Does anyone have a question for ___________?
• Can you say more about __________?
• Can someone explain __________’s strategy in your own words?
•What connections do you notice among the strategies we’ve discussed?

As you can see, the Number Talk “routine” is anything but routine. To help you think more about how to respond to students during a discussion, we particularly like Elham Kazemi and Allison Hintz’s (2014) Intentional Talk: How to Structure and Lead Productive Mathematical Discussions.

8. Number Talks don’t naturally end after fifteen minutes; often, they can go much longer if you let them—and sometimes you may want to let them. Either way, it’s useful to think ahead of time about what you might say to end the Number Talk if there are still students who would like to share their strategies. Usually, we acknowledge that we know some people still have strategies to share and express regret that we don’t have more time, and hope that the people who didn’t get a chance to share will share tomorrow.

Before you start, think about this

Number Talks turn students’ roles in math class upside down. Now they are supposed to figure something out rather than be told the steps to follow. Now they are supposed to explain what they think rather than waiting for us to explain. They also are supposed to explain why, when in the past knowing how was enough.

Now they are expected to test new ideas, with mistakes just another part of the process. Now they need to believe that their wrong answers can be opportunities rather than blemishes on their mathematical self-esteem. And the answer isn’t what matters most anymore. This is a big change for students.

But it may be an even bigger change for us, as teachers. Helping students develop these dispositions means that our roles are turned upside down, too. For those of us—and that’s most of us—who were taught that our job is to explain ideas clearly, Number Talks can feel very uncomfortable, especially at first. They may also feel at odds with what we have, in all likelihood, learned that teaching means.

Teaching as explaining is part of our cultural DNA, so it is natural to wonder how our students will adopt new strategies if we don’t first show them to students and explain why they work. Coming to believe that students can—on their own—figure out mathematically valid ways to solve problems can be both liberating and transformative for our teaching.

Learn more about Number Talks, and see many examples of teacher-student dialogue and student work, in the new book Making Number Talks Matter (Grades 4-10). You can preview the entire book online at the Stenhouse website.

_____

Cathy Humphreys is currently a doctoral student at Stanford University, where she studies mathematics teaching and learning. She has taught grades 2-12 in the California public schools for thirty years, has worked as an instructor for the Mathematics Education Collaborative and for Math Solutions, and has served as a mathematics coach for the Silicon Valley Mathematics Initiative. She is the co-author, with Jo Boaler, of Connecting Mathematical Ideas (Heinemann, 2005).

Ruth Parker is a former classroom teacher and has spent over 20 years leading professional development for math teachers in grades K-12. She is currently the CEO of the Mathematics Education Collaborative, preparing the next generation of mathematics teacher leaders for Washington state. She received her master’s degree and her Ph.D. from the University of Oregon.