A Single Open Math Task Can Work for Every Student
By Mona Iehl
Meeting the needs of every student in our classroom feels like a nearly impossible feat. However, I’ve found that facilitated open math tasks lend themselves to easily differentiating to meet all of my students’ needs.
Research tells us that, “Students’ learning is greatest in classrooms where the tasks consistently encourage high-level student thinking and reasoning and least in classrooms where the tasks are routinely procedural in nature.” (Boaler and Staples 2008; Hiebert and Wearne 1993; Stein and Lane 1996; Principles to Action, NMCT 2014).
Therefore, in order for our students to learn math with understanding, we must provide them with regular and routine opportunities to engage in problem solving and discussion of high-quality math tasks.
What are high-quality math tasks? They’ve been defined by Smith & Stein (1998) as “requiring complex and non algorithmic thinking. They require students to explore and understand the nature of the mathematical concepts, processes and relationships, and demand self-monitoring of one’s own cognitive processes….”
This means high quality tasks are not routine, procedure, memorization tasks, but instead require students to apply background knowledge without mimicking a procedure. These tasks typically have more than one right answer, draw on different skills and concepts, and ask students to analyze the context of the problem in order to choose a solution pathway.
The problem with differentiation
Differentiation can sometimes feel like just a buzzword in education. So let’s come together on a common definition of differentiation: it is tailoring instruction to meet individual needs. This could mean tailoring the content, the process, the products, or the learning environment. We support this work with ongoing assessment and flexible groupings.
Traditionally this has meant giving pre- and post- assessments, grouping students based on ability, and creating activities at varying degrees of difficulty or giving varying degrees of classroom support to achieve a common goal.
However, I would argue that when we use one high quality math task, we are able to better meet students’ needs without the alienating effects of ability grouping students or providing them with “baby work” (as my students have called simplified tasks).
We are often hesitant to give the students that struggle open math tasks. We’re afraid to give them complex tasks because we’re worried they might
- not be able to access the problem,
- fall further behind,
- get unmotivated,
- feel inferior.
However, the opposite is actually true. When we give the students who struggle “baby work” or work that is clearly grade levels lower, students end up
- missing out on opportunities to learn grade level work and fall further behind,
- feeling unmotivated because the work is “baby work,”
- feeling less than, which continues to decay their math identity.
I’ve worked in Chicago Public Schools for 15 years and for almost all of that time, I’ve taught students who struggle. I’ve witnessed time and time again that when I give students open math tasks, they can do so much more than when I give them “differentiated” tasks or too much extra support too soon.
I believe this is due to students that struggle often lacking experiences of success in math class. So in my class, when I choose a learning goal and math task with them in mind and then allow students to enter into the problem using the knowledge they do have and give them free rein to show it, they can be successful in math.
Now I will say productive struggle causes all students a bit of anxiety. However, when we use math tasks and offer students ”just in time” support, they make it over the mountain, and the confidence they show makes it all worth it.
This is truly an asset approach to learning
Open math tasks give our students an opportunity to access problems and learn from peers through sharing and discussions.
When we give students the opportunity to bring what they know to the task, they automatically have more confidence and motivation to participate. Additionally, self-efficacy is built. By giving open math tasks, we are saying to students, “We believe you have what you need inside of you to solve this problem.” Because the truth is, our students have LOTS of math understanding already!
This is what an asset approach to student learning looks like. We discover what our students do know and build on it from that point with questions and nudges to edge them along in their understanding.
Here is an example of how I differentiate a math task using the 4 steps above.
1. Choose an appropriate learning goal.
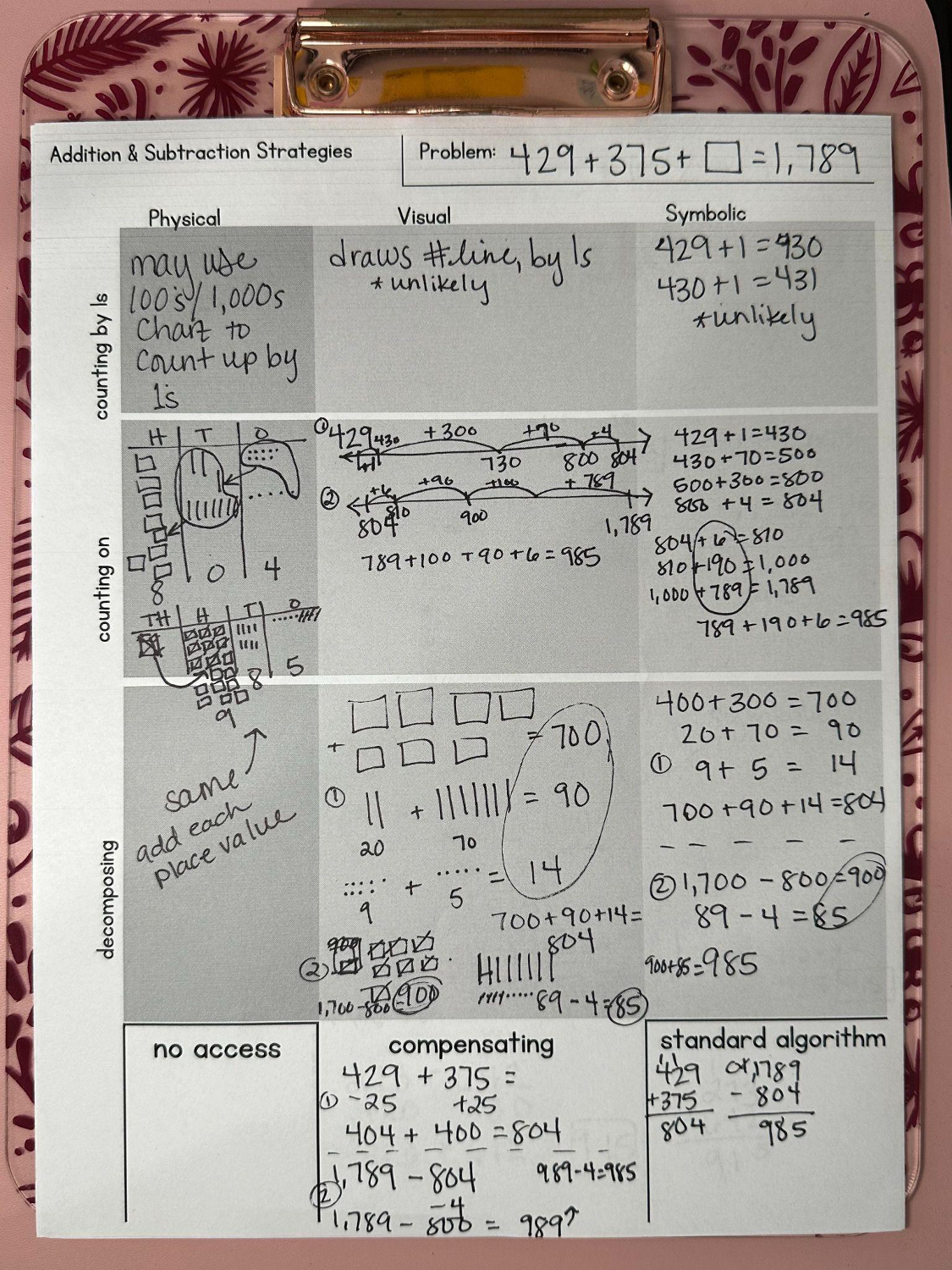
Using current, formative data from an exit ticket the previous week, I chose the goal “solve two step addition and subtraction word problems with 2 or 3 digit numbers, using strategies that show an understanding of place value and regrouping.”
I chose this goal because I noticed that many of my students could solve using the procedure of the standard algorithm, but were unable to explain the regrouping from ones to tens and tens to hundreds.
2. Choose a task that matches the goal.
I noticed in my formative data that some students were able to use algorithms that were not the standard algorithm with an understanding that demonstrated place value knowledge. I wanted to push them to apply that in a problem type that was more challenging than a typical join or separate with the result unknown.
I decided on numbers that would ask students to regroup over multiple place values in each step. I also chose to incorporate scientists observing frogs in the rainforest because it related to our current science unit to give the task a relatable context.
Here is the math task: “In the morning the scientists recorded the number of poison dart frogs they observed. They found 429 blue poison dart frogs and 375 strawberry poison dart frogs. They took a lunch break and then recorded more observations of poison dart frogs. At the end of the day they recorded 1,789. How many poison dart frogs did the scientists record after lunch?”
3. Spend time understanding how your students might solve and how their understanding might progress from basic strategies and models to more sophisticated.
I knew that some of my students were already achieving the grade level standard and were ready for a push to go deeper or explain their reasoning, while I also had students who would be modeling with manipulatives and counting by ones. I also anticipated I would have a few students who would struggle to even understand what the problem was asking. I wrote down all the ways my students might solve the problem so I could anticipate what questions and supports I would employ during the independent grapple time.
This process is called Anticipating, a term shared in 5 Practices for Orchestrating Productive Math Discussions by Stein & Smith. I recently chatted about the value of anticipating with Margaret “Peg” S. Smith on my podcast, Honest Math Chat. You can listen to that episode at MonaMath.com/podcast/80.
4. Facilitate a math discussion that allows all students to learn from one another.
I chose 2-3 students to share their ideas with the class. Through conferencing, I ensured they were prepared to discuss their ideas, and then I facilitated the sharing and discussion of their mathematical thinking.
I listened, asked questions, and nudged students’ thinking toward the learning goal. Using discussion moves, I was able to support students who weren’t able to explain the strategy and to deepen the conversation with others. (Image 2 above)
Conclusion
Using math tasks allows teachers to meet all our students’ needs while maintaining an equitable learning environment that provides access for all.
Developing math tasks to support all students’ learning shows that we:
- Trust our students enough to hold them ALL to high expectations.
- Believe they have the capability to solve complex problems.
- Are creating a classroom where students learn together to further their understanding.
That is empowered mathematics.
Mona Iehl (@HelloMonaMath) has been a fifth- and sixth-grade math teacher in Chicago, Illinois for six years. She started her 14-year year teaching career in the primary grades but found her home in the middle grades. This fall she is taking on the role of coach. For more details about her teaching methods, visit her website and see her other MiddleWeb posts here.
Mona recently took her passion for helping teachers and students find their inner mathematician to a podcast. Listen in at @HonestMathChat. More fluency resources for multiplication and division systems are available at her Mona Math TPT shop.
